It's time to create a Participation Game

29 June 2018

We are going to try and re-create the Battle of the Little Bighorn, known to the Lakota and other Plains Indians as the Battle of the Greasy Grass.  It is also commonly referred to as Custer's Last Stand.

It will be a participation game, designed to last about 30 minutes so that it is fun, fast and furious. We envisage rewards for active players and a special prize for the winner.

We will be tracking the project through its various stages over the coming months.

The battle will not be easy to re-create as the battlefield is more than three miles long and is full of ravines, hills and a wooded river runs through its length.

This was a clash between Lakota, Northern Cheyenne, and Arapaho Indians against the United States 7th Cavalry Regiment. The engagement was also partly a running battle, followed by a last ditch stand and culminated in a sort of siege.

We will be putting the project up on the Collection Calculator site so that we can account for the costs and final worth of the figures and terrain.

To make it easier we thought we would do the forces first. But this wasn't as simple as we first thought, but after some research we had found the forces involved in the battle.

They are as follows:

    7th Cavalry - Lieutenant Colonel, George A Custer

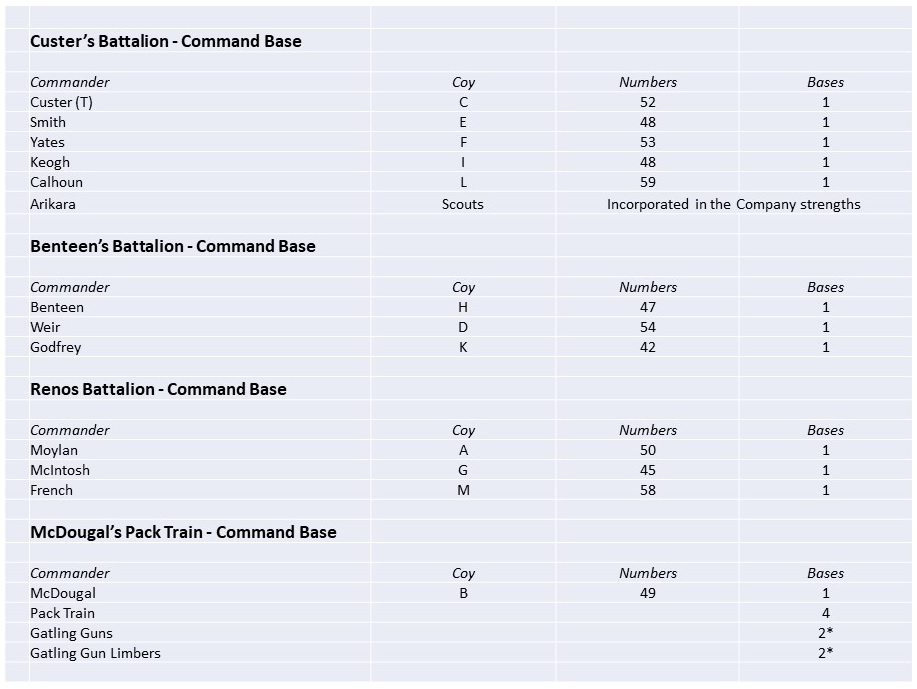
*What if they arrived with the pack train, chance.

Indian Forces - Chief Sitting Bull

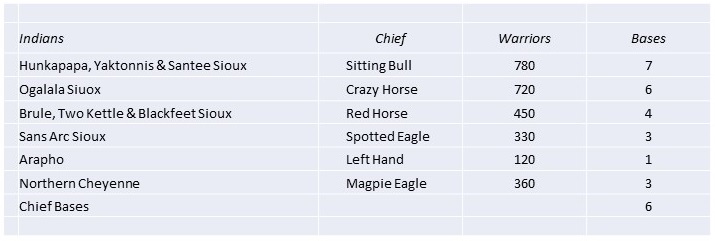
Getting started

So, now we know what forces were involved, we now need to collect the figures. This what we ordered. 

Baccus6mm Miniatures 

3 x ACW13 - Cavalry, Hat
3 x ACW14 - Cavalry, Hat, Dismounted
1 x ACW20 - CS Generals
2 x AWI01 - Indians - Bare Chested
2 x AWI02 - Woodland Indians - shirt
1 x EQU01 - Waggons
1 x EQU03 - Pack Mules
1 x CBR07 - Royal Artillery - Gatling gun
1 x ACW18 - Limbers and teams

Total cost - £82.78


Irregular Miniatures 

2 x PP4 - Red Indians mounted Braves
27 x PW1 (O) - Plains Indians light cavalry
14 x PW2 (L) - Plains Indian foot
7 x PW3 (L) - Plains Indian Command group

Total cost - £43.50 

So for an investment of only £126.28, we have all of the forces we need.

Lets us begin . . .